CHEMISTRY GLOSSARY

base-centered monoclinic lattice    →   bazno centrirana monoklinska rešetka

Base-centered or side-centered or end-centered monoclinic lattice (monoclinic-C), like all lattices, has lattice points at the eight corners of the unit cell plus additional points at the centers of two parallel sides of the unit cell. It has unit cell vectors a≠b≠c, and interaxial angles α=γ=90°≠β.

base-centered orthorhombic lattice    →   bazno centrirana ortorompska rešetka

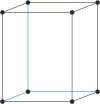
Base-centered or side-centered or end-centered monoclinic lattice (orthorhombic-C), like all lattices, has lattice points at the eight corners of the unit cell plus additional points at the centers of two parallel sides of the unit cell. It has unit cell vectors a≠b≠c and interaxial angles α=β=γ=90°.

Bravais lattice    →   Bravaisova rešetka

Bravais lattice is a set of points constructed by translating a single point in discrete steps by a set of basis vectors. The French crystallographer Auguste Bravais (1811-1863) established that in three-dimensional space only fourteen different lattices may be constructed. All crystalline materials recognised till now fit in one of these arrangements. The fourteen three-dimensional lattices, classified by crystal system, are shown to the bottom.

Crystal system	Bravais lattices
cubic a=b=c α=β=γ=90°
	simple cubic	body-centered cubic	face-centered cubic
tetragonal a=b≠c α=β=γ=90°
	simple tetragonal	body-centered tetragonal
orthorhombic a≠b≠c α=β=γ=90°
	simple orthorhombic	base-centered orthorhombic	body-centered orthorhombic	face-centered orthorhombic
monoclinic a≠b≠c α=γ=90°≠β
	simple monoclinic	base-centered monoclinic
hexagonal a=b≠c α=β=90° γ=120°
	hexagonal
rhombohedral a=b=c α=β=γ≠90°
	rhombohedral
triclinic a≠b≠c α≠β≠γ≠90°
	triclinic

body-centered cubic lattice    →   prostorno centrirana kubična rešetka

Body-centered cubic lattice (bcc or cubic-I), like all lattices, has lattice points at the eight corners of the unit cell plus an additional points at the center of the cell. It has unit cell vectors a = b = c and interaxial angles α=β=γ=90°.

The simplest crystal structures are those in which there is only a single atom at each lattice point. In the bcc structures the spheres fill 68 % of the volume. The number of atoms in a unit cell is two (8 × 1/8 + 1 = 2). There are 23 metals that have the bcc lattice.

body-centered orthorhombic lattice    →   prostorno centrirana ortorompska rešetka

Body-centered orthorhombic lattice (orthorhombic-I), like all lattices, has lattice points at the eight corners of the unit cell plus an additional points at the center of the cell. It has unit cell vectors a≠b≠c and interaxial angles α=β=γ=90°.

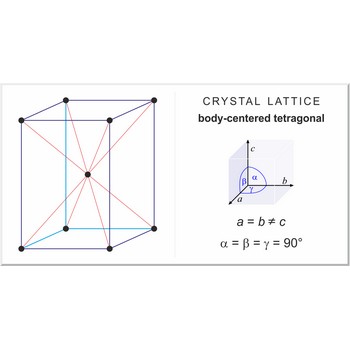
body-centered tetragonal lattice    →   prostorno centrirana tetragonska rešetka

Body-centered tetragonal lattice (tetragonal-I), like all lattices, has lattice points at the eight corners of the unit cell plus an additional points at the center of the cell. It has unit cell vectors a=b≠c and interaxial angles α=β=γ=90°.

latent heat    →   latentna toplina

Latent heat (L) is the quantity of heat absorbed or released when a substance changes its physical phase at constant temperature (e.g. from solid to liquid at the melting point or from liquid to gas at the boiling point).

lattice    →   kristalna rešetka

Crystal lattice is a three-dimensional array of points that embodies the pattern of repetition in a crystalline solid. Don’t mix up atoms with lattice points: lattice points are infinitesimal points in space - atoms are physical objects.

lattice constants    →   konstante kristalne rešetke

Lattice constants are parameters specifying the dimensions of a unit cell in a crystal lattice, specifically the lengths of the cell edges and the angles between them.

lattice energy    →   energija kristalne rešetke

Lattice energy is the energy per ion pair required to separate completely the ions in a crystal lattice at a temperature of absolute zero.